Abstract
The recently introduced trans-inductor voltage regulator (TLVR) has gained popularity in multiphase DC-to-DC applications that supply power to low voltage high current loads, such as CPUs, GPUs, and ASICs. This trend is mainly based on the outstanding transient performance of this technology. TLVR also allows design and layout flexibility but has several drawbacks. This article illustrates how TLVR design choices affect performance parameters and discusses related trade-offs.
Current Ripple and Transient in TLVR Buck
Any improvements in the multiphase buck converter are of big interest to many high current applications. Transient improvements are of particular focus, as many CPUs, GPUs, and ASICs now have very aggressive transient specifications, while a high efficiency is also critical for energy savings and thermal performance.
Current ripple in inductors is an important parameter that influences the design choices: it affects efficiency and output voltage ripple, and indirectly involves the transient performance, solution size, and other performance metrics. Another critical characteristic is a current slew rate in transient, which is a fundamental limiting factor for transient performance. Very often, current ripple (and therefore efficiency) and transient performance (direct impact on the amount of the output capacitance, etc.) cause a trade-off for the design decisions.
The conventional multiphase buck converter with discrete inductors (DL) is shown in Figure 1a. The appropriate phase shift is assumed between all phases for the optimal interleaving of the waveforms. One of the alternatives is to replace DLs with coupled inductor (CL), shown in Figure 1b.1–3,5 Another alternative is shown Figure 1c and was called TLVR, where the tuning inductor Lc affects both current ripple and transient.4,6,7,10 The TLVR approach is based on adding secondary windings to the discrete inductors and linking the phases by electrical connections of the secondary windings. This has an ideology similar to coupled inductors: averaging the AC waveforms between all the linked phases to get a better current ripple for a certain transient slew rate, but the effective coupling inductance of TLVR is limited because it has to be rated for the full phase current. The drawback is a result of the fact that the TLVR transformer does not pass the DC level of the current, so it is not canceled between the phases as it happens in magnetically coupled inductors. This article will focus on more details and particular trade-offs for TLVR, which were not possible to include to a previous study due to the paper size limitations.

Possibly, the first mathematical model with equations for ripple and current slew rate in TLVR was shown.7 While this is very helpful math that works for any circuit conditions (any duty cycle D = Vo/VIN or a number of phases Nph, etc.), it has some limitations. For example, low Lc values (tuning inductor in Figure 1c) cause increased error that becomes infinite when Lc = 0, etc. The corner with low Lc values is more critical than Lc = open corner because the main reason to use TLVR is transient improvement, which implies a reasonably low Lc value.
A more accurate TLVR derivation was shown, where the derived equation can produce the current slew rate in a steady state (for current ripple) or transient by assigning appropriate Vx states.10 The derivation was done for a more accurate equivalent TLVR schematic (Figure 2). This model has an extremely good correlation with simulations in any corner cases, but the current slew rate in a steady state is valid only for the D < 1/Nph range. The latter is acceptable because it was shown that TLVR has the maximum current ripple increase from DL baseline exactly for the D < 1/Nph region and approaches DL ripple when the Nph is sufficiently high.9,10

Typically, the TLVR value is shown in the data sheet in the same way as a discrete inductor DL, from which TLVR is derived. The model in Figure 2 assumes that TLVR total value, or the self-inductance, is split into a typically small Lk and the rest effectively becomes a mutual inductance for the TLVR transformer Lm = TLVR-Lk (Equation 1).
This current slew rate in TLVR, based on the model in Figure 2, can be expressed as Equation 2, where Lk is a TLVR leakage between the main and auxiliary windings. The Vx1 voltage is assigned to the phase of interest, while all other Vx nodes are assumed at the same voltage (VIN or 0). The corresponding node voltage Vy1 is shown in Equation 3. Equation 2 can be used for the direct calculation of the maximum transient slew rate in TLVR, forcing Vx1 = Vx and assigning these voltages to either VIN (ramping up) or 0 (ramping down). Also, the current slew rate in Equation 2 can be used for a steady state ripple calculation in Equation 4, where Vx1 = VIN and all other switching nodes are Vx = 0. Equation 4 is valid only for D < 1/Nph though, as it assumes a single and the same slew rate for the whole turn on time D/Fs.






As it was shown, figure-of-merit (FOM) is a very good indication of the system performance, and maximizing the FOM is generally a good direction to achieve the best trade-offs.9,10 Notice however that the high FOM by itself does not guarantee that every parameter in specifications for a particular application will be satisfied: high FOM is only an indicator of a good design. Defining FOM as Equation 5, which is appropriate to use for the D<1/Nph range, we can express TLVR FOM as Equation 6.
For the comparison, CL equations will be used (not shown here), while the focus will be on the TLVR performance and trade-offs.5,10 The notch coupled inductor (NCL) structure will be also used as a benchmark, compared to a particular TLVR = 150 nH solution that is footprint and size compatible.10
TLVR Trade-Offs
The key TLVR performance parameters as a function of tuning inductor Lc are shown in Figure 3, based on 12 V to 1.8 V 6-phase design (Fs = 300 kHz for the current ripple). TLVR = 150 nH is the maximum possible value to just barely meet the Isat/ph spec in a given size and therefore minimize the TLVR ripple and maximize efficiency. The DL = 150 nH is also plotted as a baseline for TLVR = 150 nH, while NCL = 6× 25 nH (Lm = 375 nH) parameters are also plotted for comparison. The actual design point Lc = 120 nH is highlighted on all TLVR curves in Figure 3.
The change in TLVR parameters needs to be considered in the content: Figure 3 shows (a) FOM, (b) current transient slew rate, and (c) current ripple as a function of Lc with the same horizontal scale. Notice that as Lc increases—all TLVR curves are asymptotically approaching the DL performance. The FOM of TLVR is increasing with lowering the Lc value, as the transient slew rate is increasing a lot, but it comes with the expense of the further current ripple increase from the already significant ripple of the DL baseline, see Figure 3c. TLVR FOM is plotted without taking into account the reduction of ferrite when the secondary winding with isolation is added to the initial DL. As expected, the TLVR ripple is always larger than the DL baseline.8–10

Figure 4 shows FOM, transient slew rate, and current ripple as a function of TLVR value (effectively Lm). It is important to notice that while mathematical curves are plotted: the Isat spec for TLVR is a full Isat per phase (Isat = 65 A for TLVR = 150 nH in the tested solution), while Isat for Lm of the NCL is significantly lower (conservative Isat = 25 A for Lm = 375 nH that has to withstand current unbalance between phases). Therefore, in the same given size of the tested solution: TLVR curves above 150 nH and NCL curves above 375 nH are only theoretical (a larger size would be needed to expand these values). As electrical models of TLVR and CL are similar, and the related curves as a function of Lm might be close to each other, the key point is that the mutual inductance in a given space will always be limited very differently for TLVR and CL.10 This puts a realistic comparison perspective between TLVR and NCL in the same specified volume.

As expected for both TLVR and NCL, an increase of Lm results in a larger coupling coefficient and a larger FOM in Figure 4a.10 The transient slew rate is generally defined by the leakage inductance Lk in NCL and the tuning inductor Lc in TLVR, not Lm, so the curves in Figure 4b are mostly flat. However, when the TLVR value (effective Lm) becomes too small—it starts effectively shorting the Lc in parallel and the transient slew rate increases rapidly.
Figure 4c confirms that increasing Lm is very beneficial for both TLVR and NCL in terms of the current ripple reduction (while Lm increase does not degrade the transient, see Figure 4b). The current ripple curves are very similar for TLVR and NCL as a function of Lm, which is expected from similarity of the electrical models, but the limits for Lm value are dramatically different.10 Of course, most of the difference comes from the required Isat rating for Lm in a given size, so the NCL has a significantly smaller current ripple than related TLVR.
Experimental Results
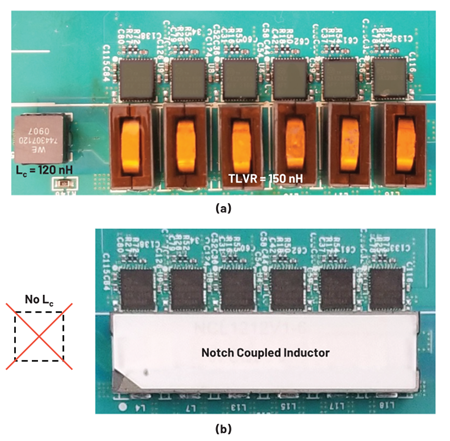
NCL was designed to fit on the same TLVR footprint and also match all other outer dimensions of TLVR solution.10 Figure 5 shows the two tested solutions on the same board (NCL does not need Lc).
Both TLVR and NCL are very fast solutions, as expected from the slew rate numbers (Figure 3b and Figure 4b). The purposely same transient performance was verified, where even lowering the Fs to 300 kHz still did not cause feedback bandwidth limitation in 6-phase solutions where phases are coupled to each other.8
As NCL has a significantly higher FOM than TLVR (Figure 3a), then matching the transient performance results in NCL having a ~2.6× smaller current ripple. A corresponding efficiency comparison is shown in Figure 6, where TLVR performance is challenged by the large current ripple peak to peak.
As leakage of CL and especially NCL is typically much lower than TLVR value, it is also expected that current capability per phase is also much higher for CL and NCL: TLVR = 150 nH example had Isat = 65 A (per phase), while NCL = 6× 25 nH in the same volume showed Isat > 300 A per phase.

Conclusion
TLVR generally has FOM~2 and from that perspective is an improvement from the discrete inductor baseline with FOM = 1. The advantage comes from the fact that TLVR improves the transient performance at a faster rate as compared to an increase in the current ripple. However, TLVR always improves only transient while creating several drawbacks. For example, TLVR current ripple is always higher than in DL with the same value due to the linking between the phases with low effective magnetizing inductance and Lc. This creates a bad efficiency impact especially considering a reduction in ferrite cross section when the secondary winding with high voltage isolation is added. The resulting additional loss of inductance value due to the loss of ferrite (assuming the same Isat as in original DL) is not considered in this article. The secondary TLVR windings connected in series also cause a potential high voltage concern, and typically result in a cost increase of the magnetic components.8
The transient current slew rate of TLVR is typically set by Lc, but if Lm is low enough: then Lm is effectively shorting Lc to make an even faster transient but with a very big current ripple punishment that affects the efficiency.
Generally, TLVR behaves similarly to a coupled inductor; however, a full current rating for TLVR limits effective Lm and makes it underperform significantly. In the same volume, CL or NCL can achieve a much higher FOM and therefore performance due to a typically several times higher Lm. As a result, NCL shows a dramatically better efficiency in the considered example, while slightly improving the transient performance of TLVR at the same time.10 This is also achieved without the cost impact or high voltage concerns of the TLVR approach.
A big advantage of Isat current capability per phase for NCL vs. TLVR comes as a bonus (>4.5× difference in example above).
References
1 Aaron M. Schultz and Charles R. Sullivan. “Voltage Converter with Coupled Inductive Windings, and Associated Methods.” U.S. Patent 6,362,986, March 2001.
2 Jieli Li. “Coupled Inductor Design in DC-to-DC Converters.” M.S. thesis, 2001, Dartmouth College.
3 Pit-Leong Wong, Peng Xu, P. Yang, and Fred C. Lee. “Performance Improvements of Interleaving VRMs with Coupling Inductors.” IEEE Transactions on Power Electronics, vol. 16, no. 4, July 2001.
4 Ming Xu, Yucheng Ying, Qiang Li, and Fred C. Lee. “Novel Coupled-Inductor Multi-phase VRs.” IEEE, 2007.
5 Alexandr Ikriannikov. “Coupled Inductor Basics and Benefits.” Analog Devices, 2021.
6 S. Jiang, X. Li, M. Yazdani, and C. Chung. “Driving 48 V Technology Innovations Forward—Hybrid Converters and Trans-inductor Voltage Regulator (TLVR).” IEEE, 2020.
7 “Multiphase Buck Converter with TLVR Output Filter.” Infineon Technologies, February, 2021.
8 Alexandr Ikriannikov. “TLVR High Voltage Considerations.” Power Systems Design, 2021.
9 Alexandr Ikriannikov. “Evolution and Comparison of Magnetics for the Multiphase DC-DC Applications.” IEEE, March 2023.
10 Alexandr Ikriannikov and Di Yao. “Converters with Multiphase Magnetics: TLVR vs CL and the Novel Optimized Structure.” PCIM Europe, May 2023.



















